It’s here! My August Tuesday book study on…
As a reminder, the schedule will be as follows…
(TODAY!) Tuesday, August 5: Discuss chapters 1-3
Tuesday, August 12: Discuss chapters 4-6
Tuesday, August 19: Discuss “A ha’s” from the remaining chapters
So let’s get started!
Chapter 1: COMPREHENSION STRATEGIES
I think that, for me, one phrase that really popped out at me in this preface was, “Good teaching is good teaching.” Nothing could be more simply stated. So often I think many teachers tend to think math as an entirely different discipline; a discipline of logic, accuracy and patterns. To a degree, this is true. However, I would argue that we ACHIEVE logic, accuracy, and patterns through a deep understanding achieved through true comprehension of the concepts–a comprehension that needs to be nurtured and coached explicitly.
If you have been in the field of education for any amount of time, you have heard the complaints that the U.S. is lagging behind in math and science. I am sure, as teachers, you have seen students come through your school without the basic mathematical understandings that you would hope for. Chapter one focuses on the strategies that we, as teachers, MUST embrace, model, and guide if we want to move our students to deeper understanding–an understanding that CAN happen with effort on our part and on the parts of our students.
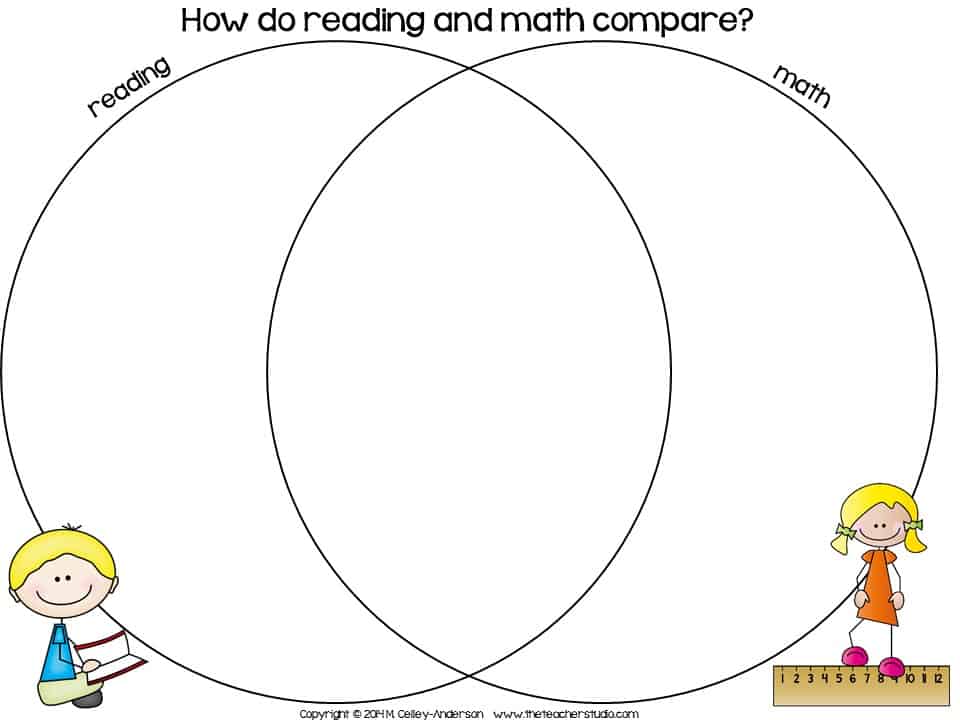
Much like in literacy instruction, there are strategies we can be teaching before our students work, while they work, and after they complete their tasks. Many of these strategies are going to require us to rethink our own understanding of math, of questioning techniques, and how we can guide our students to apply them to math contexts. I just loved reading about the way literacy and math instruction can unfold in such similar ways…won’t that make such GREAT sense to our students? In my freebie, the first page is a Venn diagram to get your students thinking about how math and reading compare. Give it a try with them!
Chapter 2: VOCABULARY
I think we all know the importance of helping students develop vocabulary in all areas of their learning. This chapter really focused on how key vocabulary is to deepening math understanding and how we, as teachers, need to tackle it through…
–Mathematical discourse
–Parental involvement
–Mathematical writing
–Math word walls
–Graphic organizers
Without providing our students with exposure to both math specific vocabulary AND more generalized word work, we are doing them a huge disservice. This chapter explains all of the above in more detail and should get your mind really turning about how to infuse more vocabulary work into your math class!
Chapter 3: CONNECTIONS
 |
| Why do we need to learn this, anyway? |
If you have taught reading, most likely you are familiar with the strategy of “making connections” or using “schema” to make better sense of texts. This strategy has always been a little worrisome to me because I have seen my own son come home with assignments like “Make 3 connections to chapter 4” or “Make a text to text connection between ___ and ___” He used to be SO frustrated because he, as a reader, did these things naturally as he read and he felt it was artificial to go back and record the thinking for “his teacher’s sake” and a grade. I also noticed students coming in making connections simply to make connections…I remember reading “Sunshine Home” by Eve Bunting with my class and a student shooting her hand in the air to make a connection. “I have a grandma too!” she exclaimed. Soon ALL the students were clamoring to tell me that they too, had grandmothers. We had to stop, pause, and think–HOW does having a grandmother help you understand the text? No one really knew. This is when I knew that our “connections” lessons were failing our readers.
The same is true with math connections! We need to make sure that our students are guided to see how these connections…
*Math to self
*Math to math
*Math to world
actually ASSIST them with their math understanding.
 |
| Is this a “crazy low” price? What does that mean? How can we tell? |
This chapter helps articulate exactly how we need to provide our students with mathematical experiences that enrich their understanding, tie concepts together, and show the real world application of these concepts. This chapter really asks us to get into the trenches with our students and help them internalize what we teach. It’s quite a challenge!
So…until next Tuesday, I hope you all had things to think about…and I would LOVE to hear your thoughts about the first part of the book. If you didn’t read–no worries. Chime in based on what I wrote above. Not sure what to say? Check out the red guiding questions below but PLEASE don’t feel tied to them!
By the way…want to grab a freebie I made that has a few “organizers” to help you apply some of these concepts from the first 3 chapters? Go ahead and grab it if you like!
So…let’s share our thoughts and reflections below. Feel free to chime in with any comments about the reading or reflect on any of the following!
1. How does the information presented in this section suggest a “shift” in your own thinking or practices?
2. What are some ways that you might integrate some of these concepts into your current practice?
3. What lingering questions and “wonderings” do you still have?