Wow…not really sure how to start this blog entry! Seriously . . . I wish I had today’s lesson on video tape! It would be so much easier (and cooler!) than trying to explain it with words. If this lesson intrigues you, it is one of the lesson in my fraction unit.
If you were with me yesterday, you know I left off with a challenge to my students–they needed to commit to either a “yes” or “no” vote on a fraction problem. Our results looked like this:
(Funny story . . . I had a sub in my room with me today –and she thought the answer was “no”. Fabulous.)
Standards for Mathematical Practice? Yes, please!
So I thought this would be the PERFECT time to review our ideas about what one of the Standards for Mathematical Practice really involves. I drew my students’ attention to one of our posters that I have reworked to be in more kid-friendly language (I have a few decorative versions of these in my store if you need something like this, but you can easily do this on your own!)
We reviewed what it would “look like” and “sound like” to critique the reasoning of others and came up with a great list of things such as be polite, use good sportsmanship, give compliments for good ideas, use a calm voice, listen to others’ ideas, and so on–and they we set to work! I used the information from the graph we made yesterday to split the class into two groups–the “Yes, it is fourths!” and “No, it is NOT fourths” and sent them to opposite sides of the room to generate evidence to support their view.
This team was very animated with their discussions and worked hard to use their diagram to prove their thinking.
Convinced he could prove his theory with measurements, this student sat off to the side collecting information!
I gave them each copies of the shape again and asked them to make sure they could use it as a tool when they were explaining. After a few minutes, I brought them back together and set them up in two teams facing each other “debate style”. (Sorry I can’t share the photo–there was no way to do it without including faces. Picture two football sidelines!)
Critique Reasoning and Justify Thinking
Pointing and explaining. . .
This student was SO adamant that they were NOT fourths. He ran over, got a whiteboard , and tried to draw another counterexample to explain that this shape wasn’t in sixths just because it was 6 pieces!
This went on for several rounds, and I didn’t feel we were making progress. No one had changed sides, and the arguments were getting somewhat repetitive. I knew I had to intervene, but I wasn’t sure how to do it without leading them in one direction or the other. So I tried this . . .
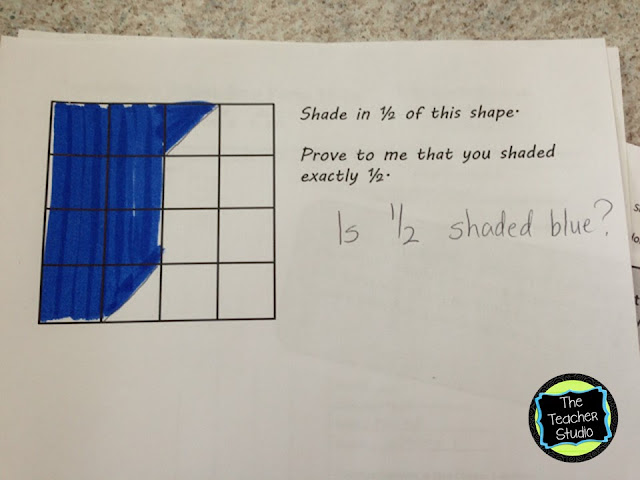
The problem states: “Shade 1/2 of this shape. Prove to me that you shaded exactly 1/2.”
They eagerly set out to shade . . .
. . . and prove
Until . . . I happened upon THIS one! Out of 22 students, only ONE divided her grid in to something other than 2 rectangles. I knew we were onto something . . .
So. . . back to the debate format we went, and I asked students to share their newly divided squares and share their thinking. We had consensus that each 1/2 needed to have 8 squares. I purposely did NOT let the little petunia in the photo above share until several others had . . .
When she did, she spoke quite eloquently and said something along the lines of, “I knew that 1/2 of the grid had to be 8 of the 16 squares. All of you put all 8 of your squares together to make a rectangle, but they didn’t HAVE to be! I made a different shape and it is still 1/2 because it is still 8 out of the 16 squares!”
Imagine the pause. I let the silence sink in for a few minutes. All of a sudden, the chatter started up full force and two “no” students joined the “yes” team. This same student asked if she could explain how she could use this idea to prove why the other diagram really WAS in fourths. I could hardly contain myself when I shrugged nonchalantly and said, “Sure.” I was ready–I could almost feel the other “no” kids ready to bolt to the “yes” side!
Explaining her thinking . . .
Using the Smartboard to talk about equal sides and not needing to be the same shape…
She explained beautifully. . . AND NO ONE MOVED! The “no” team’s argument? You cannot compare the two problems because the grid problem had 8 squares but THEY WERE ALL THE SAME SHAPE–the other problem had squares and triangles, and they were NOT the same at all!
I could NOT believe it! NOW what was I going to do? I was sure she had found the missing piece that would help them see the light. So, once again, I had to think on my feet. I grabbed a copy of the grid and colored one to look like this and threw it under the document camera.
I figured this was my last hope. I asked the class if I had shaded 1/2 of the grid blue–and noted that not all of the shapes were the same. . . some shaded areas were squares and others were triangles.
2 more came to the “yes” team. Seriously–only TWO! My students are SO stubborn!
I figured I probably had one more attempt before either abandoning ship or simply telling them the answer. So I asked one of my most surly “no” team members how he would define “fourths”.
“Something divided into four equal pieces,” he said confidently. He then reminded me that our diagram was most definitely NOT divided into four equal pieces (followed by moans from the “yes” team!).
Foiled.
I asked another member (The one with the ruler above–convinced that there was a half millimeter of error somewhere) of the “no” squad to define fourths a different way. He thought and then said . . .
“A fourth is a half of a half.”
Silence.
5 kids moved to the “yes” team. Several other “yes” members team members then worked together to explain how “That’s what they meant!” . . . that the original shape was divided in 1/2–and then each 1/2 was divided in half again. . . . that fourths didn’t mean four shapes of the same SHAPE, but four shapes of the same AMOUNT.
Victory. The rest of the students move over . . . but I’m still not convinced one of them (white board guy) really believes it. I think tomorrow I’ll have him do some cutting and pasting of squares and triangles to play with it a little more.
I then followed up with another activity about representing 1/2 in different ways, but that might have to wait for another day.
So . . . we spent 60 full minutes on this investigation today and it was exhausting and exhilarating all at once. I absolutely loved watching the students process and reprocess and refine their thinking. Their homework tonight? I quickly created another grid–this time a 5 x 5 grid and asked them to represent 1/2 of it and prove it to me for tomorrow. This should be interesting! Thanks for continuing to follow our fractions saga as I try to make the Common Core come alive in my room. Tomorrow is Friday–enjoy it!